4A Homework Set 7

Center of mass and momentum conservation

1. A length of rope L of total mass M is suspended at rest just above a digital balance. As the rope is released, find the reading of the pan balance as the rope falls onto the balance as a function of time.

2. A block of mass m₁ slides along a frictionless table to the right with a speed of v₁. A second mass M₂ slides in the same direction with a speed of v₂ such that it will collide with m₁ which is in front of m₂. Say that a spring of stiffness k is attached to one of the masses such that it compresses when the two masses finally collide. Find the maximum compression of the spring.

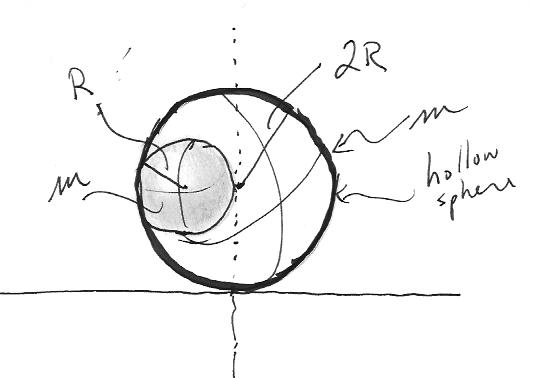
3. A ball of mass m and radius R is placed inside a larger hollow sphere with the same mass and inside radius 2R. The combination is at rest on a frictionless surface in the position shown in the diagram. The smaller ball is released, rolls around the inside of the hollow sphere, and finally comes to rest at the bottom. How far will the larger sphere have moved during this process?



4. A dog of mass M is standing on a raft so that he is a distance L from the shore. He walks a distance d on the boat toward the shore and then stops. The boats has a mass of M_b. Assume no fricton between the boat and the water. How far is the dog from shore when he stops moving?.

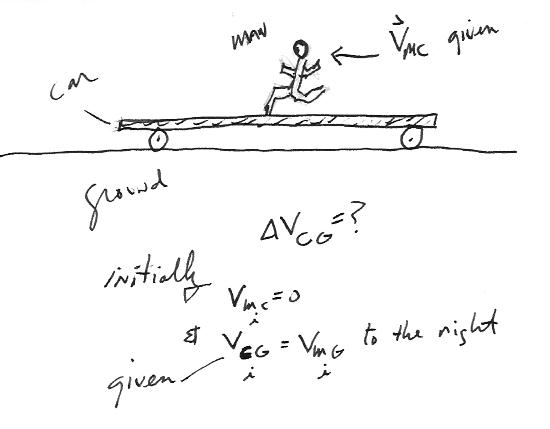
5. A railroad flatcar of mass M_c can roll without friciton along a straight horizontal track as shown in the diagram. Initially a man of mass M_m is standing on the car which is moving to the right relative to the ground with a speed of v_i. What is the change in velocity of the car relative to the ground if the man runs to the left so that his speed relative to the car is given as v_mc just before he jumps off at the left end?



6. A ball with mass M moving horizontally at a speed v, collides elastically with a block of mass 3m that is initially hanging at rest from a celing on the end of a wire of length L. Find the maximum angle through which the block swings after it is hit.

7. A 3 kg body moving at 4 m/s makes an elastic collision with a stationary body of mass 2 kg. Find the velocity of each body after the collision.

8. Find the center of mass position for a half circular disk. Uniform density. Radius R and total mass M.

9. A 20 kg projectile is fired at an angle of 60 degrees above the horizontal with a speed of 80 m/s. At the highest point of its trajectory, the projectile explodes into two fragments with equal mass, one of which falls vertically with zero initial speed. How far from the point of firing does the other fragment strike if the terrain is level?