Equipment List:
Introduction:
In this lab, you will conduct an experiment to calculate the rotational inertia of an object (the disk or the hoop) and then compare it with a theoretical value.
As the hanging weight falls,
the rotating platform starts to spin. The gravitational potential energy
between the earth and hanging mass decreases and the translational kinetic
energy of the hanging mass and the rotational kinetic energy of the rotating
platform both increase. The total energy is conserved if there is no external
work done by friction to decrease the system's energy.
Theory:
Derive an equation that gives
the rotational inertia of the rotating object (that is, the spider and anything
else that may be resting on it) in terms of the hanging mass, the height
through which it falls, the time through which it falls, and the radius of
the "drum" of the spider where the string is wrapped around it. One way is
to use the work-energy theorem and kinematics.

Procedure:
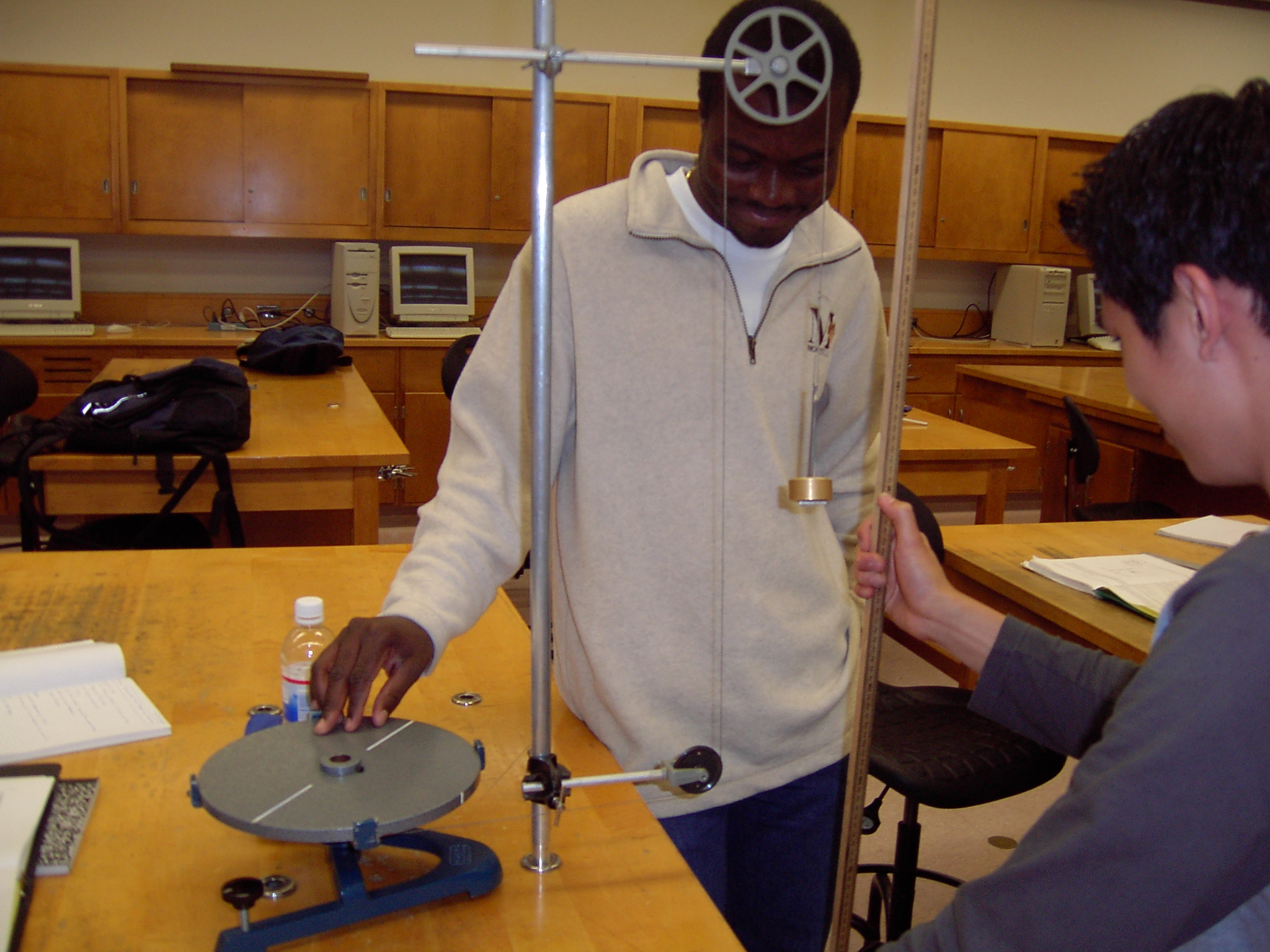
1. Set up and align your apparatus
as shown.
2. You will need to measure the radius of the drum of the rotating platform.
Use the vernier calipers.
3. Measure the hanging mass with the digital balance. Use one hundred
to two hundred grams, including the hanger.
4. A run will consist of letting the hanging mass fall from rest through
a distance measured with the two meter stick (the distance should be as long
as possible, at least
one meter). Measure the time it takes to fall through this distance.
5. Do five runs with just the spider alone (no disk or hoop on the spider)
to calculate the total rotational inertia of the entire system (the spider
and the two pulleys). Find the average value of this "intrinsic" rotational
inertia and its uncertainty using the statistical method.
6. Now run the experiment five more times but with the disk on the rotating
platform. Calculate the "new" rotational inertia (this would be the rotational
inertia of the disk and the "intrinsic" rotational inertia of the
system. Use the statistical method to find the average value and its uncertainty.
7. To calculate the theoretical value of the disk's rotational inertia,
you will need to measure the radius of the disk. The mass of the disk is too
large to measure on the balance, so for its mass, use the value that is etched
on the disk's face.
Calculations:
The experimental value of the disk's rotational inertia is calculated by
subtracting the intrinsic rotational inertia from the "new" rotational inertia.
Using uncertainty propagation, find the uncertainty in the experimental value.
That is, since the experimental rotational inertia for the disk (or hoop)
is found by a subtraction (the subtraction of the total rotational inertia
of the spider and disk together by the rotational inertia of just the platform),
then the uncertainty in the subtracted value is found by adding the uncertainties
of the total and the platform in quadrature.
Using the well known formula for the rotational inertia of a disk (or
hoop) rotating about its center of mass, calculate the theoretical value.
Use uncertainty propagation to calculate its absolute uncertainty. Treat
the given mass etched on the disk as exact and only use the uncertainty in
your measured radius of the disk to find the uncertainty in its rotational
inertia.
Analysis:
Compare the two values. Do their most probable ranges overlap? Calculate
a descrepancy test between the two average values. You should get less than
ten percent or you might be making a mistake. Less that five percent is good.
Knowing that systematic errors are present from dissipative forces, would
you expect your experimental value to be greater or less than the theoretical
because of those errors? Why?
Repeat the whole thing with the hoop if there is time. Consult your instructor
A refinement: By letting the hanging mass bounce at the bottom of its path (but not hitting the floor!) and travel back toward its initial position, you can estimate the energy loss within the system and compensate for the systematic error it introduces. By noting the difference in height between where the hanging mass initially started and how close it returns after bouncing, the energy loss can be estimated.